Supplement 3.2: Plane and Solid Angles (2/2)

The Solid Angle

Solid angles come about when the concept of plane angles is extended into the three-dimensional space. Hence, a plane circle (2D) turns into a sphere (3D); the length of an arc of a circle (2D) turns into the surface area of a sphere (3D). The solid angle Ω as shown in the illustration below corresponds to the ratio of the sphere's segment area a to the square of the sphere's radius R:

Ω = \frac{a}{R^{2}}

A solid angle Ω within a sphere. It is equal to the ratio of the sphere's segment area a to the square of the sphere's radius R. The segment area is depicted as a circle on the surface of the sphere; however, there are no limitations as to form and shape of solid angles.

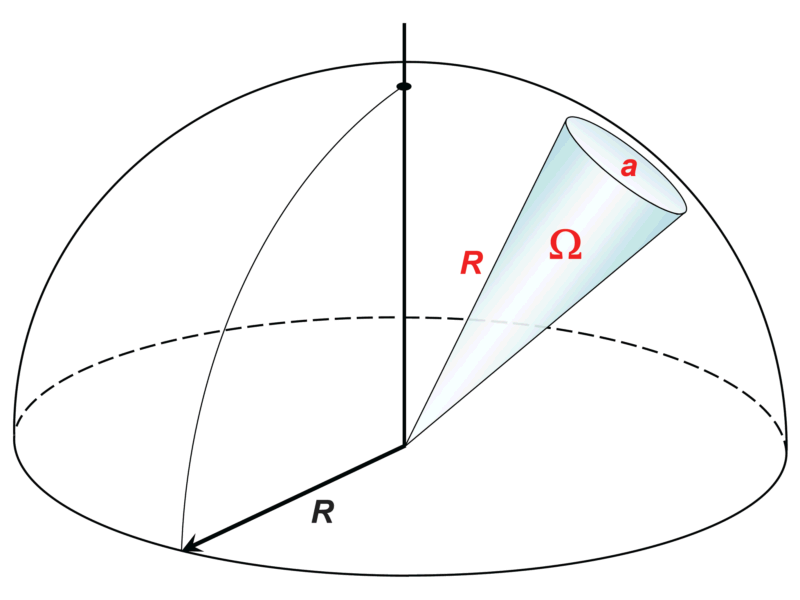
Quantities of solid angles are expressed in dimensionless units called steradians with the symbol sr. Quantities in degrees such as in plane angles are not used.

Problem: Solid angles ↓

Using the equation for the surface of a sphere, compute the solid angle arising out of the following physical quantities of the spheres's segment area a:
a) surface area of a hemisphere (half of a sphere, see illustration above)
b) surface area of a full sphere

The firmament from horizon to horizon (on flat country where no mountains interrupt the firmament) is one example for the solid angle of a hemisphere.

The results of your computations should be: a) $2 π$ , b) $4 π$ .

For small solid angles, the segment area of a sphere can be approximated to be considered as a flat surface, thereby simplifying the computation of solid angles.

Differential solid angles

Just as a plane angle can be expressed as a differential quantity,

d φ = \frac{d s}{r}

so can solid angles likewise be expressed as differentials:

d Ω = \frac{d a}{R^{2}}

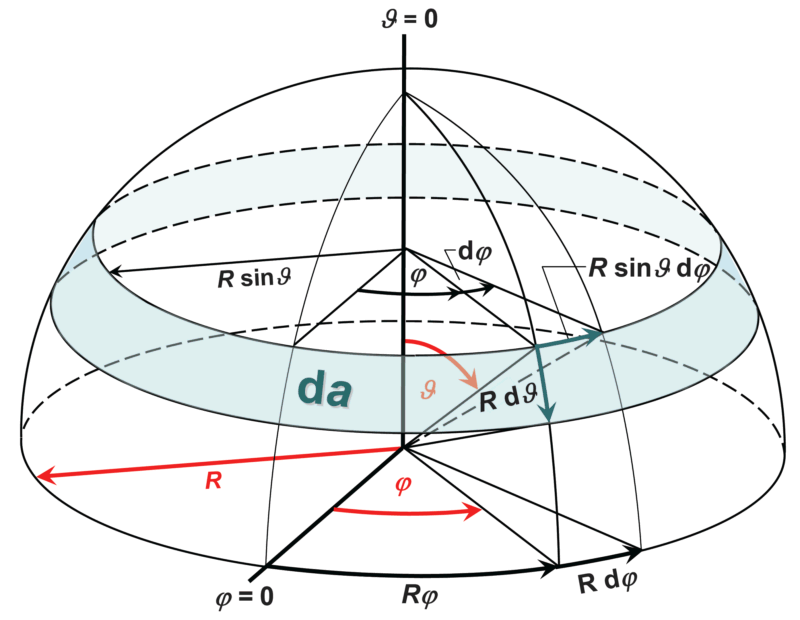
The orientation of a solid angle element in space is best given in spherical coordinates (or: polar coordinates). The illustration on the right shows a differential segment area da of a sphere in the polar coordinates (r,ϑ,φ); by dividing this by the square of the sphere's radius R you get the differential solid angle dΩ.

A differential segment area da on a spherical surface. Divide this by the square of the sphere's radius R and you get the solid angle dΩ.

Given the geometrical relations that apply for the edge of the differential segment area da assumed as a flat rectangle in polar coordinates (see illustration above), the solid angle can be expressed as:

d Ω = \frac{d a}{R^{2}} = \frac{(R sin ϑ d φ) (R d ϑ)}{R^{2}} = sin ϑ d ϑ d φ

Many physical processes are mainly dependent on the zenith angle ϑ, whereas the azimuth angle φ is irrelevant. Therefore, the variable φ in the equation for da can be eliminated by integration:

\int_{φ = 0}^{2 π} (R sin ϑ d φ) (R d ϑ) = R^{2} sin ϑ d ϑ \int_{φ = 0}^{2 π} d φ = 2 π R^{2} sin ϑ d ϑ

The resulting differential segment area da is similar to a belt having the width R dϑ winded around the sphere just below the zenith angle ϑ (see illustration below).