Supplement 4.8: Een experiment met de lichtverstrooiing van water

Lichtverstrooiing door water, een voorbeeld van Rayleigh-verstrooiing

De absorptiecoëfficiënt van vloeibaar water... is minder dan 1/m voor alle zichtbare golflengten. In het blauwe bereik is water bijzonder transparant, daar is de absorptiecoëfficiënt ongeveer 0,02/m.

De verstrooiingscoëfficiënt van water is ongeveer een factor 10 kleiner in het blauwe gebied. Dit komt door de geringe grootte van het molecuul in vergelijking met de golflengte van het licht: de diameter is ongeveer 2 Å of 2·10^-10 m (de Ångström, met het eenheidsteken Å, is een gangbare lengte-eenheid in de atoomfysica). Watermoleculen zijn dus een voorbeeld van deeltjes die worden gekenmerkt door Rayleigh-verstrooiing.

Hier onderzoeken we hoe een watermonster het licht van een laser verstrooit.

De experimentele opzet

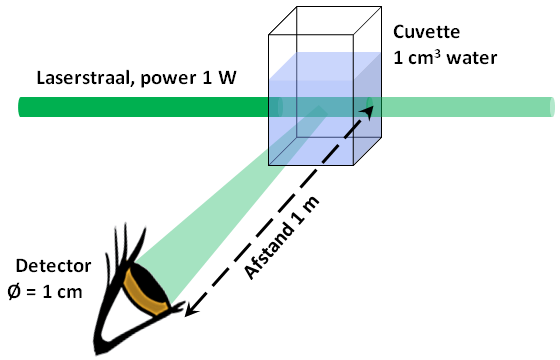
Een met water gevulde cuvet wordt verlicht met een blauwgroene laserstraal. De golflengte is 500 nm, het stralingsvermogen is 1 W (als u het experimenteel wilt nabootsen: deze laser is absoluut niet oogveilig, wees uiterst voorzichtig).

Onder een hoek van 90° tegen de richting van de laserstraal in, nemen we eerst met het oog het verstrooide licht waar dat op het water wordt geproduceerd. Laat het verlichte en zichtbare watervolume V = 1 cm³ bedragen.

Experimentele opstelling voor de meting van de lichtverstrooiing van een watermonster.
Bron: Rainer Reuter, Universiteit van Oldenburg, Duitsland.

Vervolgens bevestigen we een fotodiode voor een kwantitatieve meting op een afstand van r = 1 m van de cuvette en ook onder een hoek van 90°. Laat de diameter van het lichtgevoelige gedeelte d = 1 cm. zijn. Laat de gevoeligheid van de fotodiode bij 500 nm, d.w.z. de fotostroom (in ampère) van de diode bij een optisch vermogen (in watt), gelijk zijn aan S = 0,5 A/W.

Verstrooiingseigenschappen van water

Zeer kleine deeltjes verstrooien het licht symmetrisch in de voorste en achterste helft van de ruimte, dit is kenmerkend voor de Rayleigh-verstrooiing van kleine deeltjes. Als de verstrooiingshoek gemeten ten opzichte van de richting van de laserstraal straal wordt aangeduid met θ, wordt de verstrooiingsfunctie voor ongepolariseerd licht gegeven door de vergelijking

β (θ) = β (90 °) (1 + {cos}^{2} θ)

Polair diagram van de fasefunctie versus hoek θ voor deeltjes met de diameter 2 Å, met de brekingsindex n = 1,33 en voor licht met golflengte 500 nm. De fasefunctie is gelijk aan de verstrooiingsfunctie β gedeeld door de verstrooiingscoëfficiënt b.
Gemaakt met MieSimulatorGUI, versie 1.3, laatste toegang: 30 Maart 2023.

The literature value of the scattering function for the scattering angle 90° at the wavelength 500 nm is (Zhang & Hu, 2021):

β (90 °) = 1.38 \cdot 10^{- 4} \frac{1}{m sr}

The molar mass of water is about 18 g, correspondingly the molar volume is about 18 cm³. The number of particles in the mole is given by the Avogadro constant 6·10²³. The number of water molecules in 1 m³ is then:

N = \frac{6 \cdot 10^{23}}{18 \cdot 10^{- 6} m^{3}} = 3.3 \cdot 10^{28} \frac{1}{m^{3}}

The differential cross-section of the scattering at 90° results in:

σ (90 °) = \frac{β (90 °)}{N} = 4.14 \cdot 10^{- 33} \frac{m^{2}}{sr}

The brightness at the eye

The intensity of the scattered light follows from the values of the scattering function β of the water and the irradiance E of the laser on the illuminated water volume V according to

I = β E V

where E = 1 W/cm² and V = 1 cm³. The optical power at the eye or detector results from this intensity multiplied by the solid angle Ω at which the eye or detector appears as seen from the scattering water:

P = I (90 °) Ω = β (90 °) E V \frac{π d^{2} / 4}{r^{2}}

Substituting all values gives the optical power P ≈ 10^-10 W or 0,1 nanowatt. Can the eye still see this?

In order to assess this, it is useful to go over to the photon image of light. A dark-adapted eye can perceive about every 50th photon striking the retina as a faint flash of light. For monochromatic light, the relationship between optical power and the corresponding photon number is as follows:

P = N_{P h o t o n} E_{P h o t o n}

where N_Photon is the number of photons per second, and the photon energy E_Photon = hc/λ. h is the Planck constant and c is the light velocity. It follows N_Photon ≈ 250· 10⁶ or 250 million photons per second.

The signal of the detector

The photocurrent of the photodiode results from the incident optical power and from its sensitivity, which has been assumed to be S = 0.5 A/W above. It becomes:

I_{p h o t o} = S P = 0.5 \cdot 10^{- 10} A

or 50 picoampere. Electrical multimeters only reach into the microampere range at best, so you need a much more sensitive ammeter. Such devices are called picoammeters.

The scattering coefficient

If the scattering function is integrated over the entire space, the scattering coefficient results:

\begin{array}{l} b = \int_{Ω = 4 π} β d Ω = 2 π \int_{θ = 0}^{π} β (θ) sin θ d θ \\ = 2 π β (90 °) \int_{θ = 0}^{π} (1 + {cos}^{2} θ) sin θ d θ = 16 \frac{π}{3} β (90 °) \end{array}

Inserting gives: $b = 2,3 \cdot 10^{- 3} /m$

Over a distance of 1 m, the laser beam loses 2.3 per mille of its brightness due to scattering. The total cross-section of the scattering cross-section (integrated over the entire room) becomes:

σ = \frac{b}{N} = 7 \cdot 10^{- 32} m^{2}

compared to their geometric cross-sectional area, the molecules thus only shadow a very small part of the brightness of the laser beam.

n fact, there are some additional influencing variables in the light scattering of water. Temperature, pressure and - in the case of seawater - salinity play a role. And density fluctuations occur in the liquid, which also contribute to the scattering. The publication of Zhang & Hu, 2021 provides detailed information on this.