Supplement 3.3: Measuring Radiance

Radiance

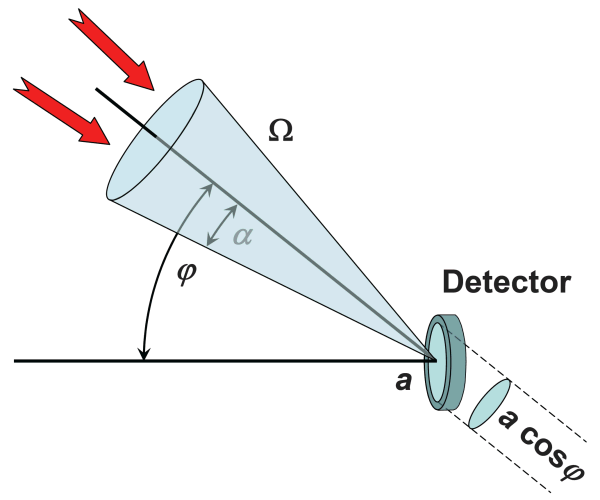
Radiance is a radiometric quantity used to describe the propagation of light and radiation. It is indicated by the symbol L and is equal to the optical power P being emitted out of a small solid angle dΩ falling on a small surface da (a radiation detector, for instance):

In a measuring instrument, differentials are replaced by finite quantities: a detector's sensitive surface a measures the optical power P coming out of the solid angle Ω. Radiance data are then computed out of these quantities, based on the calibration of the instrument.

The detector's sensitive surface a receives light rays coming out of a solid angle Ω, in this example falling at an angle φ on the surface normal of the detector. The projection of the surface a towards the direction of the solid angle is a cosφ.

The output signal of a detector is a current or voltage - depending on the physical effect being used - which is then converted into an optical power by calibration. The size of its sensitive surface can either be measured or obtained out of the technical specifications.

The solid angle from which the detector receives the signal is often unknown and usually quite large, so that it is necessary to narrow down and define it quantitatively by using other optical elements. The following paragraphs describe how this is done.

Radiometers measuring the radiance from the sky and from the water surface. From the data, the daylight reflectance of the water surface can be calculated, which provides information on suspended particles in the water.

Defining the solid angle with an aperture

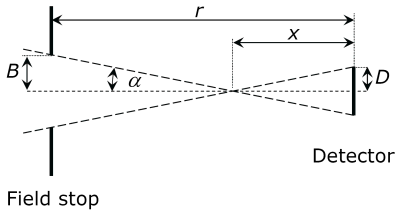
The simplest way to limit the visual field of a detector is by using an aperture.

     

A detector with an aperture to limit its visual field.

Detector and aperture are at a distance r from one another. The detector's radius is D, the aperture's radius is B. α corresponds to the biggest possible acceptance angle in half.

Defining the solid angle with lens and aperture

The efficiency of a detector with aperture is very poor. Much higher efficiencies can be achieved when adding a collecting lens to the set-up.

     

A detector with a collecting lens and an aperture for limiting the field of view.

The maximum acceptance angle α is defined by the lens (with radius L and focal length f) and the aperture at distance b. The sensitive area of the detector behind the aperture is assumed to be sufficientlly large so that the detector does not limit the path of rays. The acceptance angle α follows from the image of the aperture B' at a distance b' from the lens.