1. Light and Radiation
Electromagnetic waves (1/4)
The title of this paragraph suggests that light waves have to do with electricity and magnetism. This is indeed one of the most prominent findings of classical physics in the 19th century. How did it all happen?
- Electricity has been known since the time of the ancient Greeks. The word electron comes from the Greek word for amber, ηλεκτρον. Rubbing a peace of amber with a fur produces electric charges which attract feathers and can create sparks when brought close to a grounded object. Also magnetic minerals were known which attract iron objects.
- In 1820, the Danish physicist Hans Christian Ørsted discovered a connection between electricity and magnetism: an electric current in a wire can deflect a compass needle from its usual orientation. Hence, electric currents give rise to magnetic fields around the wire which exhibit forces to magnetic objects. This was the advent of electrodynamics.
- In 1831, the English physicist Michael Faraday found that a moving magnet induces an electric current in a wire. He could show that varying magnetic fields cause electric fields around the magnetic field lines.
- Finally in 1856, James Clerk Maxwell made an attempt to bring all the earlier findings together into a single theory. Maxwell's equations explain electric charges as the source of electric fields, while magnetic charges do not exist. Instead, magnetic fields are caused by electric currents or time-varying electric fields. Similarly to the latter, time-varying magnetic fields induce electric fields. This appears very complex. But the mathematical formulation is straightforward, yielding equations which are very symmetric with respect to the electric and magnetic field. They are considered to be amongst the most beautiful equations in physics! We need differential and integral calculus, which is eventually beyond the scope of this tutorial. You will learn about it in physics class, or you read more about Maxwell's equations in supplement 1.1.
What has all this stuff got to do with electromagnetic waves? Well, remember that electric charges and currents bring about electric and magnetic fields. As it appears, there are no such fields in the absence of charges and currents. There is an exception: coupled time-varying electric and magnetic fields can exist in the absence of electric charges and currents! These coupled fields are called electromagnetic waves.
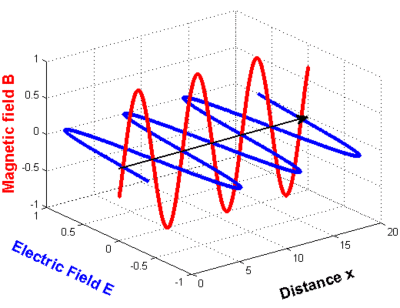
One can show with Maxwell's equations that the electric and magnetic field vectors are always perpendicular to each other. Both are perpendicular to the direction of wave propagation, and therefore electromagnetic waves are transverse waves.
The transverse character of electromagnetic waves is depicted in the graph below.
The graph above shows a plane monochromatic wave which is the most elementary example of a wave. Have a look at a propagating wave in an animation. What is a plane monochromatic wave?
- Plane waves propagate in one direction only, here: along distance x.
- Monochromatic waves have one value of the wavelength and period (and hence frequency) only, as already shown on the previous page.
Obviously, waves with these ideal properties are difficult to realise. Daylight or the light from lamps consist of a mixture of waves having different wavelengths and amplitudes, and their path of rays is divergent. Almost ideal plane monochromatic waves can be produced with lasers. The principles of lasers and the characteristics of laser radiation are discussed in a SEOS tutorial entitled Remote Sensing using Lasers.